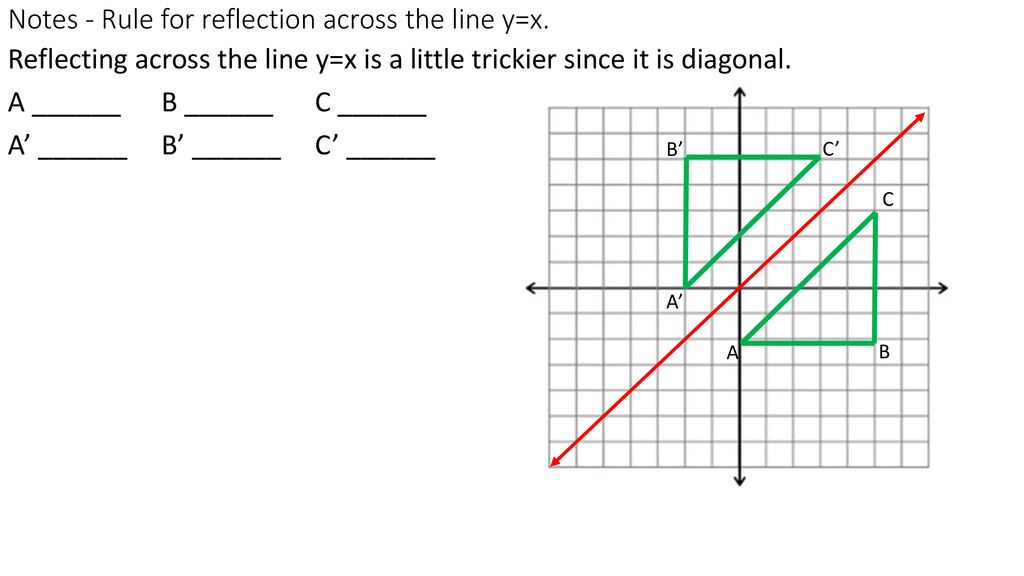
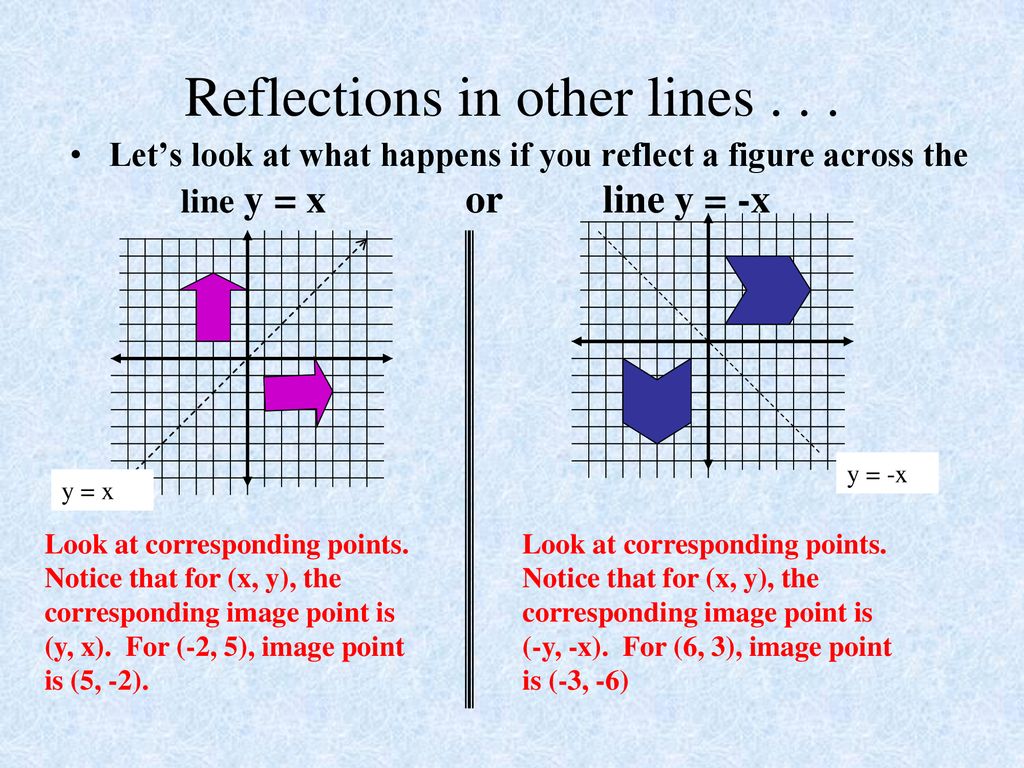
Reflection over the line y = x A reflection in the line y = x can be seen in the picture below in which A is reflected to its image A' The general rule for a reflection in the y = x (A, B) → (B, A)Report an issue Q Which statement correctly identifies the line of reflection?C figure C A clock is constructed using a regular polygon with 60 sides The polygon rotates every minute How much has the polygon rotated after 7 minutes?

Reflections In Math Formula Examples Practice And Interactive Applet On Common Types Of Reflections Like X Axis Y Axis And Lines
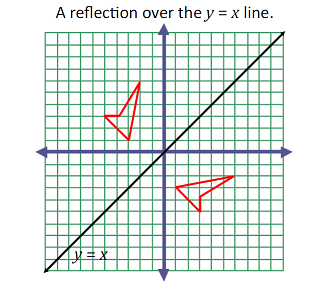
Reflection across the line y=x graph
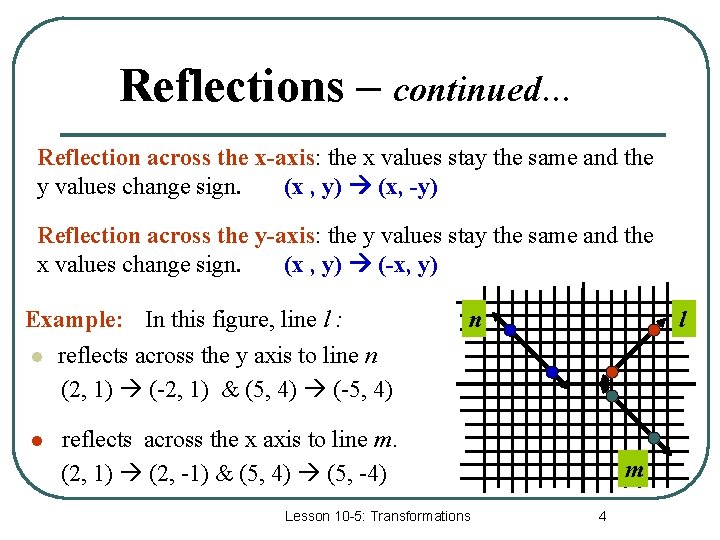
Reflection across the line y=x graph-When you reflect a point across the line y = x, the xcoordinate and ycoordinate change places If you reflect over the line y = x, the xcoordinate and ycoordinate change places and are negated (the signs are changed) the line y = x is the point (y, x) the line y = x is the point (y, x) Reflection in a Line A reflection over a line k (notation r k) is a transformation in which each point of the original figure (preimage) has an image that is the same distance from the line of reflection as the original point but is on the opposite side of the line Remember that a reflection is a flip Under a reflection, the figure does not change size



Q Tbn And9gctvjthqkdwbpqfxmfukfqyrhm8wtlbtfnfu2u0osqggs0pct8ms Usqp Cau
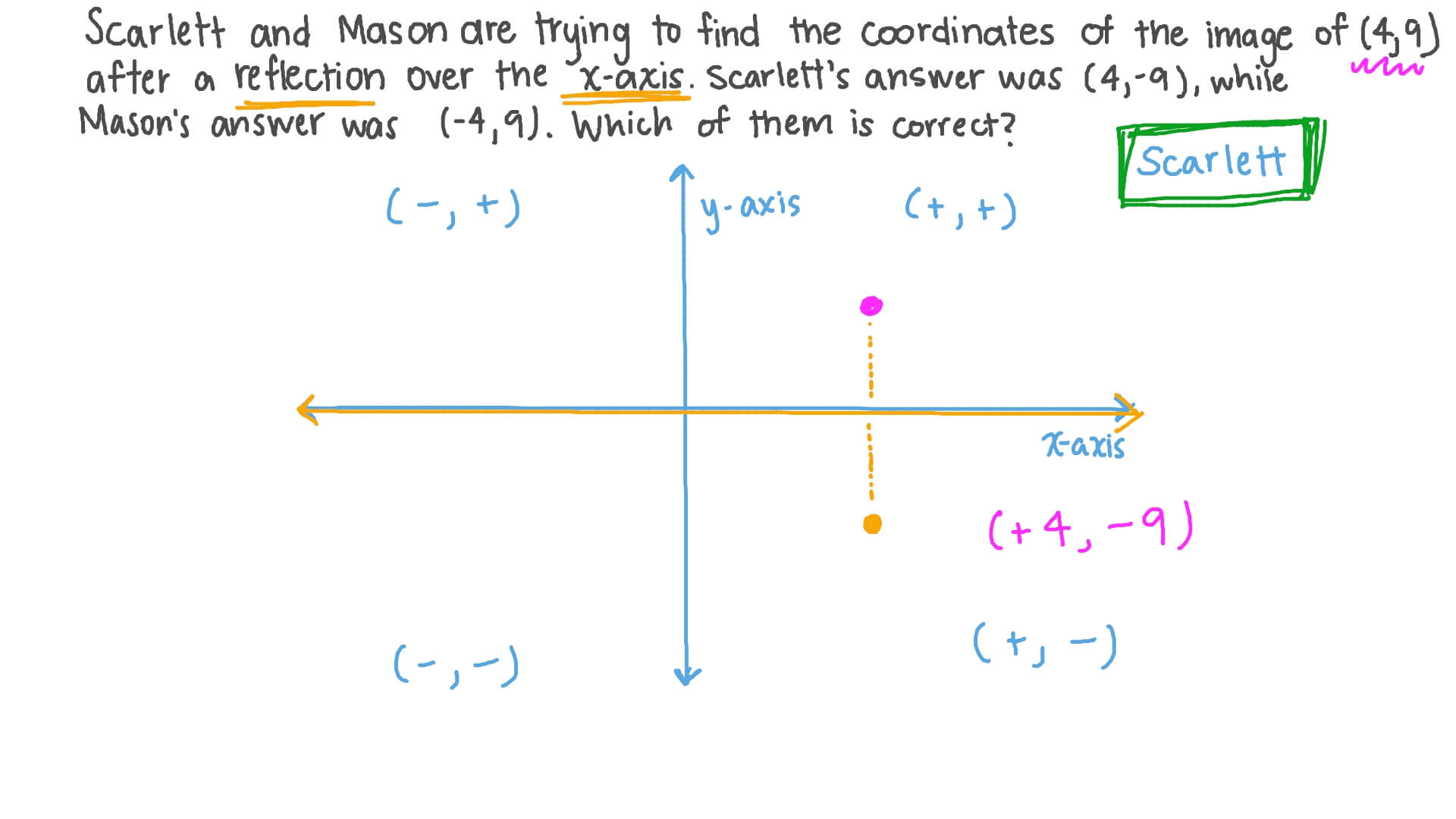
Stack Exchange network consists of 177 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack Exchange 🔴 Answer 3 🔴 on a question What are the coordinates of the image of vertex f after a reflection across the line y = x?This video demonstrates how to reflect a figure over the line y=x It shows two methods of reflecting over y=x The video shows how to count towards the y=x
Reflection about the line y=x Once students understand the rules which they have to apply for reflection transformation, they can easily make reflection transformation of a figure For example, if we are going to make reflection transformation of the point (2,3) about xaxis, after transformation, the point would be (2,3)This is a KS3 lesson on reflecting a shape in the line y = x using Cartesian coordinates It is for students from Year 7 who are preparing for GCSE This page includes a lesson covering 'how to reflect a shape in the line y = x using Cartesian coordinates' as well as a 15question worksheet, which is printable, editable and sendableThis lesson is presented by Glyn CaddellFor more lessons, quizzes and practice tests visit http//caddellpreponlinecomFollow Glyn on twitter http//twitter
Reflect over the y = x When you reflect a point across the line y = x, the x coordinate and y coordinate change places If you reflect over the line y = x, the x coordinate and y coordinate change places and are negated (the signs are changed) The reflection of the point (x,y) acrossQuestion options A) Reflection across the line y = – x B) Reflection across the line y = x C) Reflection across the origin D) Reflection across the xaxis Question 7 5 / 5 points A point (–7, –6) is rotated counterclockwise about the origin to map onto (–6, 7) The angle of rotation is Question options A) 270° B) 90° C) 180Reflection over the line y = x Which rigid motion maps A(3, 1) to A'(1, 3)?



Assignment 2 Transforming Parabolas



What Is The Reflection Over The Y Axis Of The Point 4 7 Quora
Answer choices The triangles are reflected across the xaxis The triangles are reflected across the yaxis The triangles are reflected across the line y = x The triangles are reflected across the line y = x Reflection Across Y=X When reflecting over the line y=x, we switch our x and y, and make both negative Reflection Over Y = X In order to define or describe a reflection, you need the equation of the line of reflectionReflections A reflection is a transformation representing a flip of a figure Figures may be reflected in a point, a line, or a plane When reflecting a figure in a line or in a point, the image is congruent to the preimage A reflection maps every point of a figure to an image across a fixed line The fixed line is called the line of reflection



Answered Write The Coordinates Of The Vertices Bartleby



Solution Describe The Relationship Between The Graph Of Y 2x 1 And It 39 S Reflection Across The Line Y X
See the answer See the answer See the answer done loading Show transcribed image text Expert Answer Who are the experts?Q Reflect over xaxis Which point would be at (3, 6) Q Which axis is the horizontal (leftright) one?If (a, b) is reflected on the line y = x, its image is the point (b, a) Geometry Reflection A reflection is an isometry, which means the original and image are congruent, that can be described as a "flip" To perform a geometry reflection, a line of reflection is needed;




Ixl Reflections Find The Coordinates Grade 7 Maths Practice




Reflection Over Y 2 With Rule Educreations
Usually you should just use these two rules T (x)T (y) = T (xy) cT (x) = T (cx) Where T is your transformation (in this case, the scaling matrix), x and y are two abstract column vectors, and c• (1,3) 0 (3,1) (1,3) (3,1) g(23) f(13) e 1,5) h(25) the answers to answerhelpercomA Condition of Reflection when Y = X Take the case where a point is reflecting across a line Y=X Now, the X and Y coordinates will interchange their positions However, the signs get negated/cancelled when the point of reflection takes place over a line Y = X, but the point of coordinates still changes places (Image to be added soon)



Question Video Determining The Coordinates Of A Point After Its Reflection Over The 𝑥 Axis Nagwa




Reflection Rules How To W 25 Step By Step Examples
Finding the inverse from a graph Your textbook probably went on at length about how the inverse is "a reflection in the line y = x"What it was trying to say was that you could take your function, draw the line y = x (which is the bottomleft to topright diagonal), put a twosided mirror on this line, and you could "see" the inverse reflected in the mirrorWhen you reflect over xaxis the coordinates are (x,y) and when you reflect over the yaxis the coordinates are (x,y If you want to reflect over y=x then the coordinates are (y,x) If you want to reflect over y=x the coordinates are (y,x) Comment on Caylen Jang's post "You can use aWhich figure represents the image of parallelogram LMNP after a reflection across the line y = x?



Reflection Translation Reflection Rotation High School Geometry Unlocked 16




Reflection Rules How To W 25 Step By Step Examples
How do you reflect lines? Stepbystep explanation Under a reflection in the line y = x a point (x, y ) → (y, x ) , thus (8, 1 ) → ( 1, 8 ) kason11wd and 4 more users found this answer helpful heart outlined Thanks 3 star starGeometry 3 people liked this ShowMe Flag ShowMe Viewed after searching for reflect over x= 1 reflection over the line y=x Reflection over y=x reflection over yaxis reflection



Reflection Definition Reflection In The Coordinate Plane



Unit 6 Day Ppt Download
23 Questions Show answers Q Flipping a figures is a Q Is the picture being reflected in the yaxis or xaxis? reflection across the line y=x second blank answer choices reflection across the xaxis reflection across the yaxis rotation 90 clockwise about the origin translation 4 units to the right Answers 2 Get Other questions on the subject Mathematics13 Another way To reflect along a line that forms an angle θ with the horizontal axis is equivalent to rotate an angle − θ (to make the line horizontal) invert the y coordinate rotate θ back Further, y = mx implies tanθ = m, and 1 m2 = 1 cos2θ Then, assumming you know about rotation matrices, you can write




How To Reflect A Graph Through The X Axis Y Axis Or Origin




Reflect The Square Across The Line Y X Followed By Chegg Com
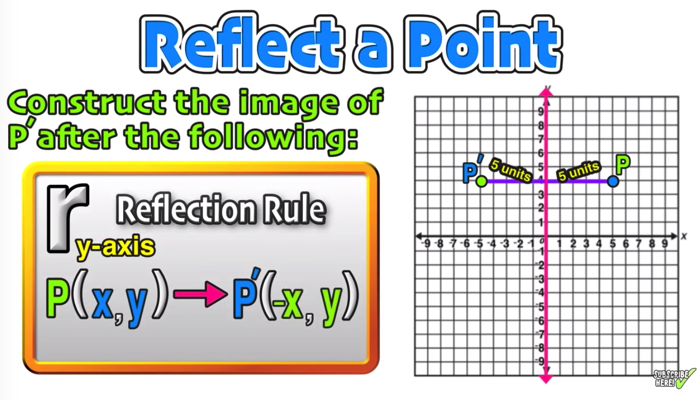
Reflection Transformations in 2Space Let such that and suppose that we want to reflect across the axis as illustrated Thus the coordinate of our vector will be the opposite to that of our image The following equations summarize our image Thus our standard matrix is , and in form we get that Of course there are other types of reflection0 (4,4) O (4,0) O (04) (44) This problem has been solved!Use our online point reflection calculator to know the point reflection for the given coordinates This calculator helps you to find the point reflection A, for the given coordinates of A(x,y) Just select an axis from the dropdown and enter the coordinates, the point reflection calculator will show the result




Transformations Boundless Algebra



Great Minds Eureka Math Blog
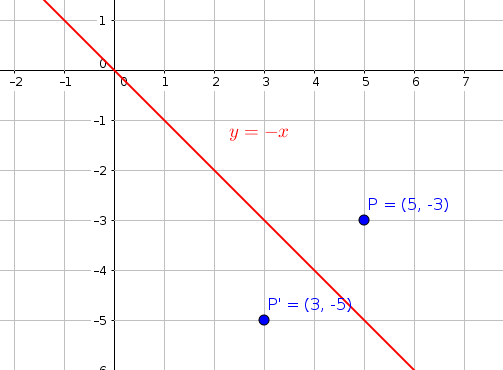
File previews pdf, 975 KB Worksheet for students to use to reflect shapes in the x and y axes Also reflecting in the line y=x (diagonal line bottom left to top right) Tes classic free licence Report this resource to let us know if it violates our terms and conditions Our customer service team will review your report and will be in touchThere are at least two ways of doing so Method 1 The line y = 3 is parallel to xaxis Let the required image is P′ By common sense, we know (Distance between the line y = 3 and point P) = (Distance between line y= 3 and point P′) Since line join If one reflects a figure across the yaxis, the points of the image can be found using the pattern (x, y) ⇒ (–x, y) Taking the result from the first reflection (x, –y) and applying the second mapping rule will result in (–x, –y), not (y, x), which reflecting across the line should give



Www Westerly K12 Ri Us Site Handlers Filedownload Ashx Moduleinstanceid 2346 Dataid 66 Filename Unit 1 2 review key Pdf




How To Graph Reflections Across Axes The Origin And Line Y X Video Lesson Transcript Study Com
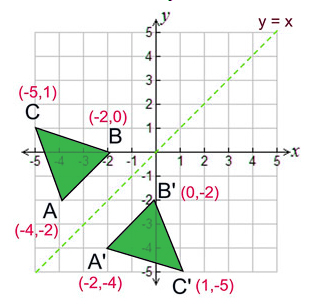
The rule for reflecting over the X axis is to negate the value of the ycoordinate of each point, but leave the xvalue the same For example, when point P with coordinates (5,4) is reflecting across the X axis and mapped onto point P', the coordinates of P' are (5,4)The resulting orientation of the two figures are oppositeThe line that represents y=x has a slope of 1/1 If i am considering a point, ill refer to it as A at (4,3) and reflect it over line y=x i will be at (4,3) which i will refer to as point B I can prove this relationship using simple geometry I wi



Q Tbn And9gctvjthqkdwbpqfxmfukfqyrhm8wtlbtfnfu2u0osqggs0pct8ms Usqp Cau




Which Point Would Map Onto Itself After A Reflection Across The Line Y X Maps Location Catalog Online
Question Which point would map onto itself after a reflection across the line y = x?90˚ counterclockwise rotation about the origin OR 270˚ clockwise rotation about the origin What is #A'#, the image of #A(3,5)#, after a reflection in the line #y = x#?



What Does It Mean To Reflect Over The Y X Line Quora




Reflection Transformation Matrix
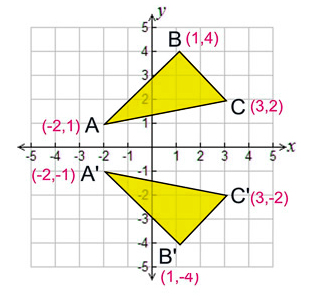
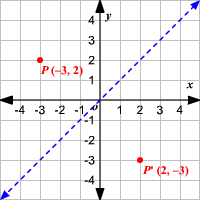
Find the image of the point (3, 2) that has undergone a reflection across a) the xaxis, b) the yaxis, c) the line y=x, and d) the line y=−x Write the notation to describe the reflection Solution 39A shape can be reflected in the line y = −x If point on a shape is reflected in the line y = −x both coordinates change sign (the coordinate becomes negative if it is positive and vice versa) the xcoordinate becomes the ycoordinate and the ycoordinate becomes the xcoordinateA reflection across the line y = x switches the x and ycoordinates of all the points in a figure such that (x, y) becomes (y, x) Triangle ABC is reflected across the line y = x to form triangle DEF Triangle ABC has vertices A (2, 2), B (6, 5) and C (3, 6) Triangle DEF has vertices D (2, 2), E (5, 6), and F (6, 3)




Reflections In Math Formula Examples Practice And Interactive Applet On Common Types Of Reflections Like X Axis Y Axis And Lines




Geometry Transformations
Precalculus 1 Answer Jim G image A' = (5 , 3 ) Explanation Any point (x , y ) when reflected in the line y = x has an image ( y , x ) example A (3 , 4 ) has image A' ( 4 , 3 ) Answer link Related questions When a figure is reflected over the line y=x, notice that the coordinates change order We can also reflect a figure in the coordinate plane in any point on the coordinate plane Here's a dart reflected in the point (12,10) The point the dart is reflected over is called, you guessed it, the point of reflectionA reflection is a "flip" of an object over a line Let's look at two very common reflections a horizontal reflection and a vertical reflection




Reflection Transformation Matrix



What Is The Image Of 2 5 Reflected Across X 2 Socratic
From the diagram we see the object point ( − 2, −5) is mapped to (x',y') by a reflection in the line X = 2 we note (1) the ycoordinate is unaffected (2) for reflections the distance from the line of reflection to the object is equal to the distance to the image point ∴ a = 2 2 = 4units so the image point is 4 units from the line ofDefining key concepts ensure that you can accurately define key ideas, such as the three types of reflections across the y or x axes, around the origin, or across the y=x lineGet the free "Reflection Calculator MyALevelMathsTutor" widget for your website, blog, Wordpress, Blogger, or iGoogle Find more Education widgets in WolframAlpha




Reflections In Math Formula Examples Practice And Interactive Applet On Common Types Of Reflections Like X Axis Y Axis And Lines




Ixl Reflections Graph The Image Geometry Practice
(a) Write the equation of a line that intersects the negative xaxis and the positive yaxis at points not equidistant from the origin (0, 0) (b) Draw the line (c) Draw the line that is the reflection of your line across the line y=x (d) Find the equation of the line drawn in Part (c) Do not convert fractions, if any, to decimals



Reflection Over The X And Y Axis The Complete Guide Mashup Math



How To Reflect A Graph Through The X Axis Studypug



Solution What Is The Image Of A 3 1 After A Reflection First Across The Line Y 3 And Then Across The Line X 1



Reflections




Reflection



Reflection Notes Videos Qa And Tests Grade 9 Optional Mathematics Transformation Kullabs



1




Reflection Mathbitsnotebook A1 Ccss Math



View Question Reflection Across The Line Y X




Reflecting Points Video Reflections Khan Academy




Answered Rules For Reflections On A Coordinate Bartleby



What Is The Reflection Image Of 5 3 In The Line Y X Socratic
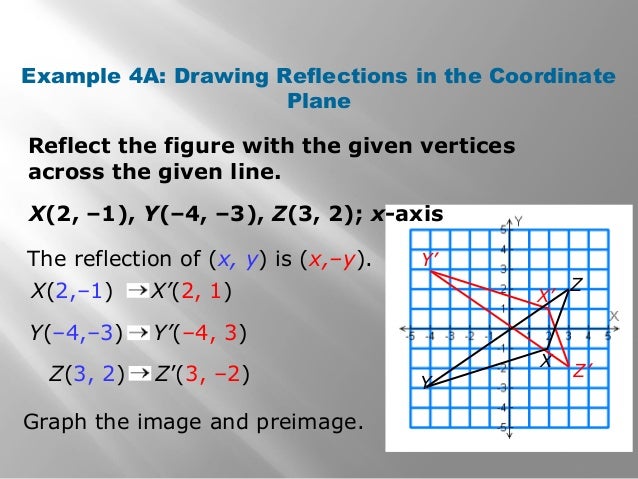



Geometry Unit 9 2




How To Find A Reflection Image
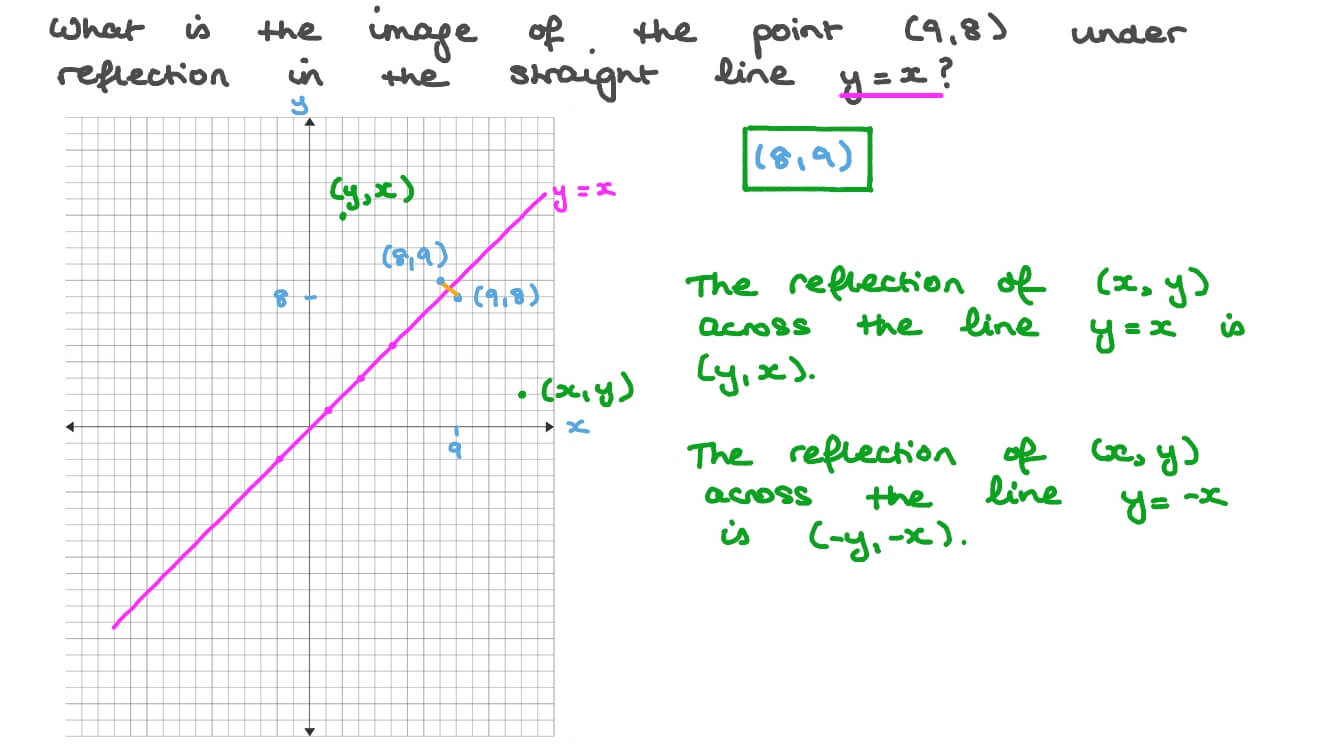



Question Video Determining The Position Of A Point After Reflecting In A Given Straight Line Given The Point S Coordinates Nagwa




Reflection Rules How To W 25 Step By Step Examples



Biomath Transformation Of Graphs



Solved Graph A Triangle Label It Lmn And Reflect It Over The Line Y X To Create Triangle L M N Describe The Transformation Using Words Draw Course Hero




Reflections Geometry Abroad




Reflection Mathbitsnotebook A1 Ccss Math




Reflections Across Y X Geogebra




Write The Coordinates Of The Vertices After A Reflection Over The Line Y 3 Brainly Com




In The Xy Coordinate Plane Point P Is The Reflection Of The Point With Coordinates 3 1 Across The Line Y X Point T Is The Reflection Of Point P Across The Y Axis What




In The Figure Shown Triangle Rst Undergoes Reflections Across Two Line R S T Is The Final Brainly Com
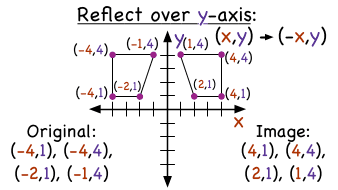



How Do You Use Coordinates To Reflect A Figure Over The Y Axis Virtual Nerd



1
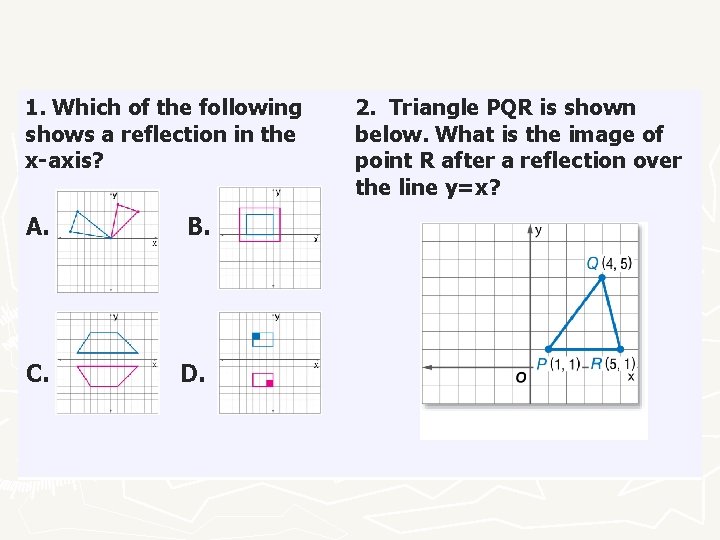



Warmup Reflect Triangle Abc Across The Line Y



Transformations Dilations Translations Reflections Rotations Ppt Download




Reflections In Math Formula Examples Practice And Interactive Applet On Common Types Of Reflections Like X Axis Y Axis And Lines




Reflection Mathbitsnotebook A1 Ccss Math




What Is The Image Of 6 4 After A Reflection Over The Line Y X Homeworklib




Transformations Of Graphs




Reflections Ck 12 Foundation




Reflection Transformation Matrix



Geometric Reflections Q35 50 Intro To Geometry




Learn About Reflection Over The Line Y X Caddell Prep Online



1



Http Www Dentonisd Org Cms Lib Tx Centricity Domain 11 Chpt9notes Pdf



Lesson Transformations Lesson 10 5 Transformations 1 Types




Reflection In Geometry Examples Solutions Videos Worksheets Games Activities




Graphs Of Reflections Ck 12 Foundation




Reflection In The Line Y X Geogebra




Reflection Over The Y X Line Youtube



Illustrative Mathematics
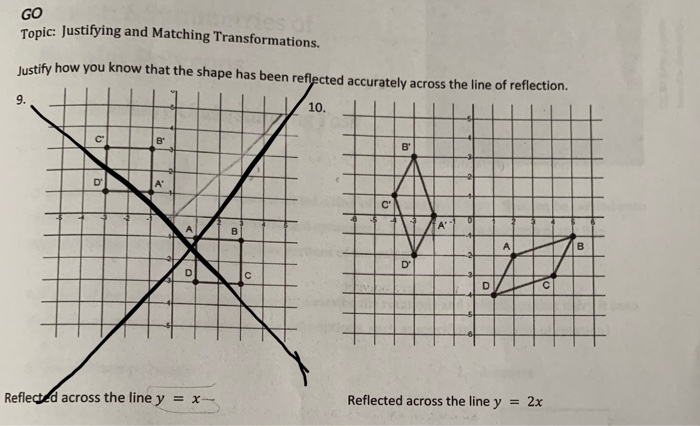



Go Topic Justifying And Matching Transformations Chegg Com



Reflection Definition Reflection In The Coordinate Plane



Why Aren T Reflected Lines Perpendicular Meaning Why Are Their Slopes Negative Not Negative Reciprocals Enotes Com




How To Find A Reflection Image



Reflection Across The Line Y X Geogebra




Reflection Over The X And Y Axis The Complete Guide Mashup Math




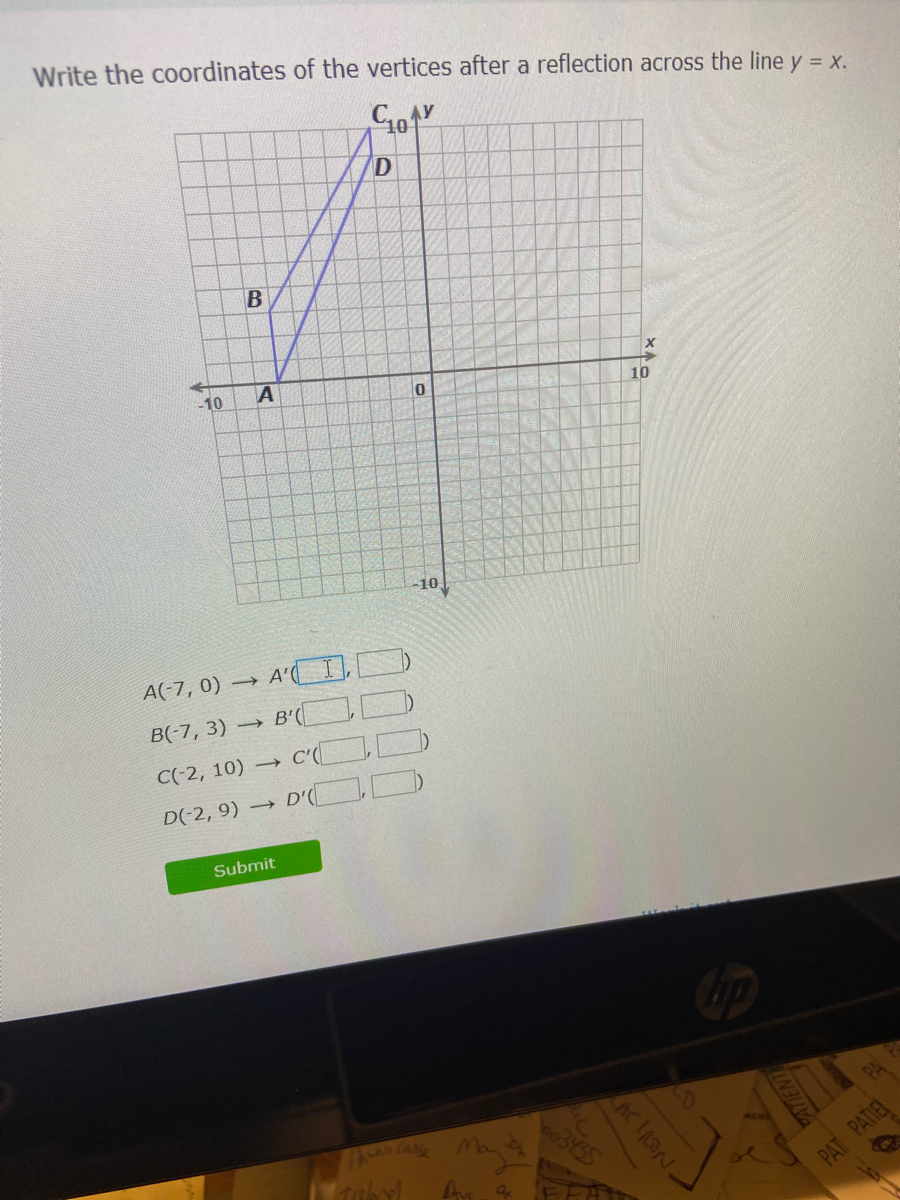
Write The Coordinates Of The Vertices After A Reflection Across The Line Y X Brainly Com



Http Www Laureli Com Uploads 1 2 2 3 09 15relections Pdf




Reflection Over Y X Math Geometry Showme
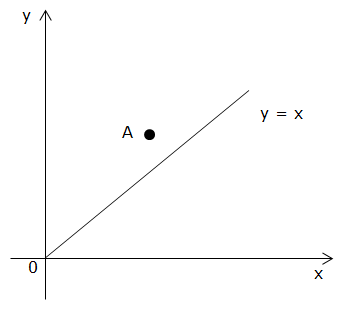



In The Rectangular Coordinate System Above The Line Y X Ttp Gmat Blog




Transformation Reflection Over The Line Y X Youtube



Chapter 8 Page 460
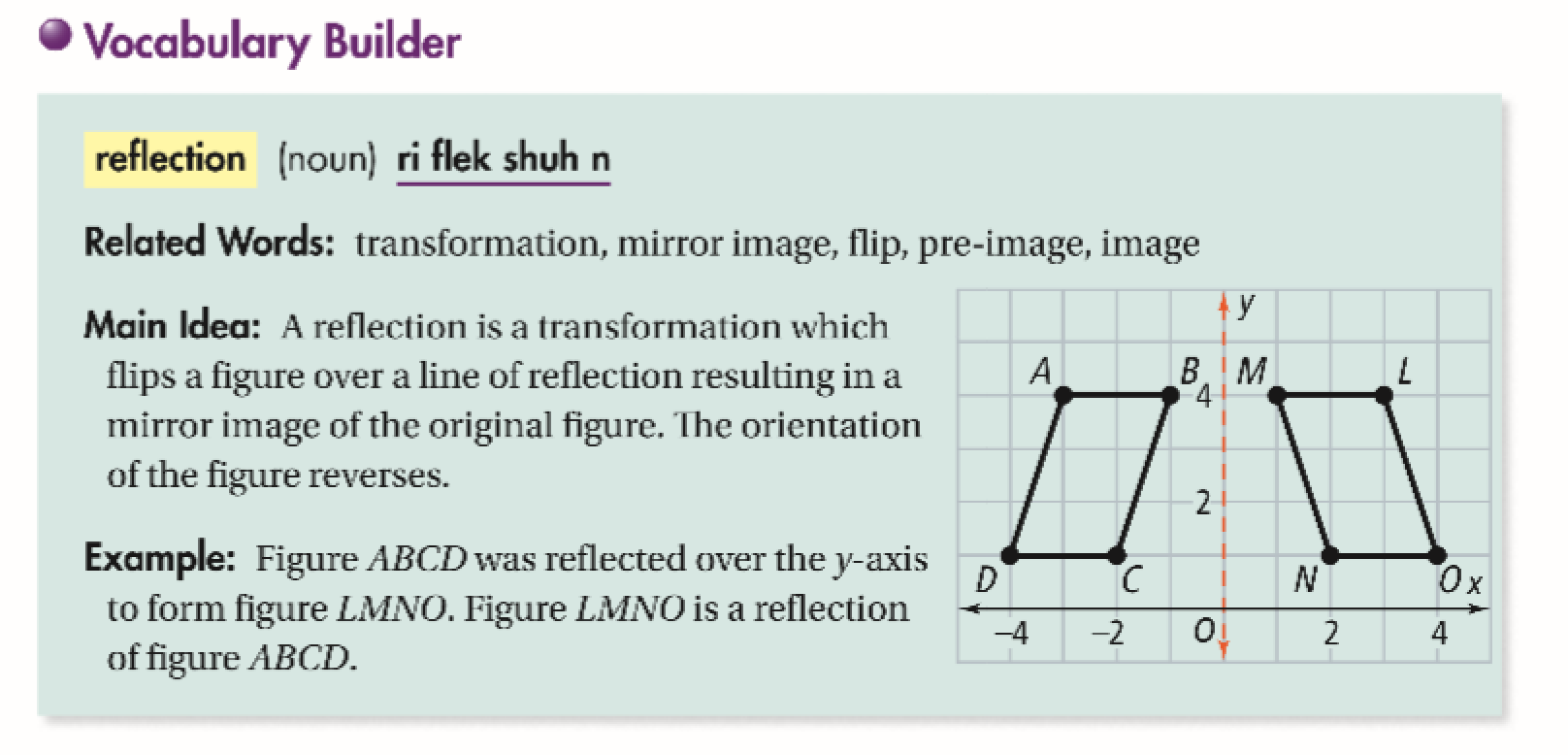



Reflections Across A Line Other Than Axis Or Y X Power Jasmin Library Formative




Reflection Mathbitsnotebook A1 Ccss Math




How To Graph Reflections Across Axes The Origin And Line Y X Video Lesson Transcript Study Com



1 Geometry Ms Singarajah 7



Image Point Coordinates After Reflection Across Any Line Bluevelvetrestaurant




How To Reflect A Graph Through The X Axis Y Axis Or Origin




Reflection Definition Reflection In The Coordinate Plane




Reflect The Square Across The X Axis Followed By A Chegg Com




Reflection Mathbitsnotebook A1 Ccss Math



Linear Reflections Across X And Y Axis Youtube




Reflections Interactive Worksheet By Natalie Belcher Wizer Me




Picture Of Reflection Across Y Axis Reflection Math Reflection Math
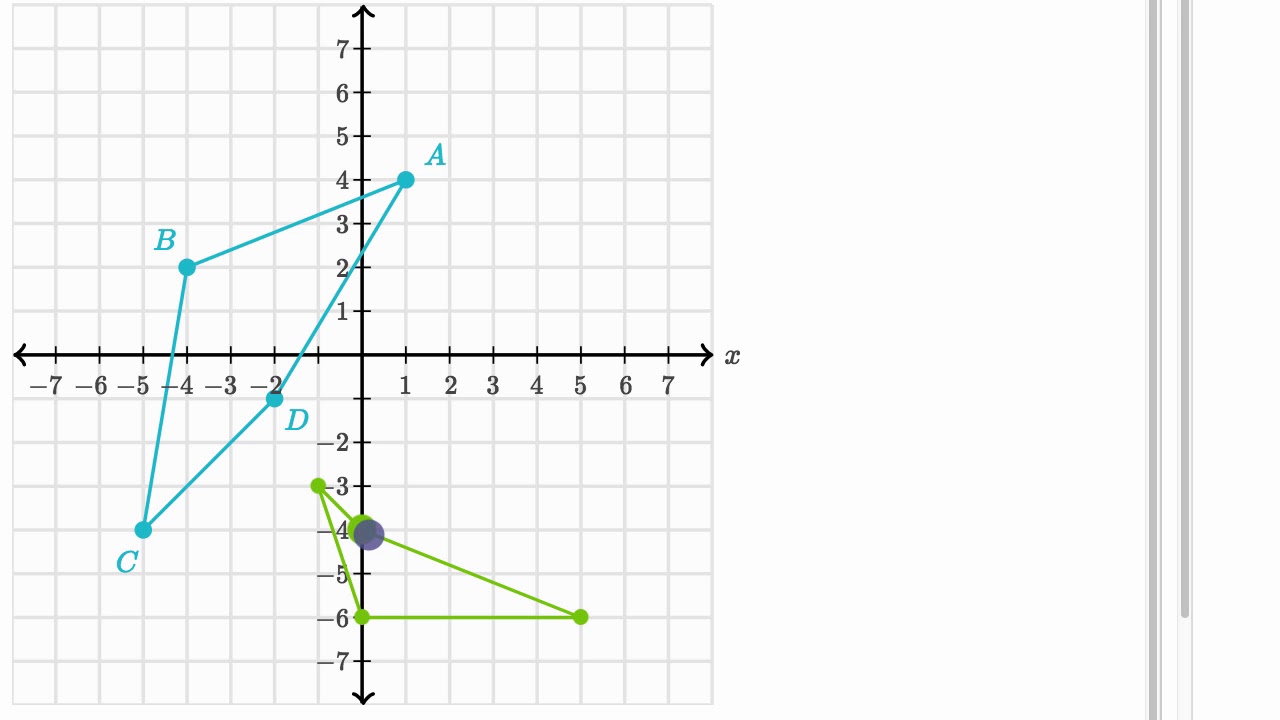



Reflecting Shapes Video Reflections Khan Academy
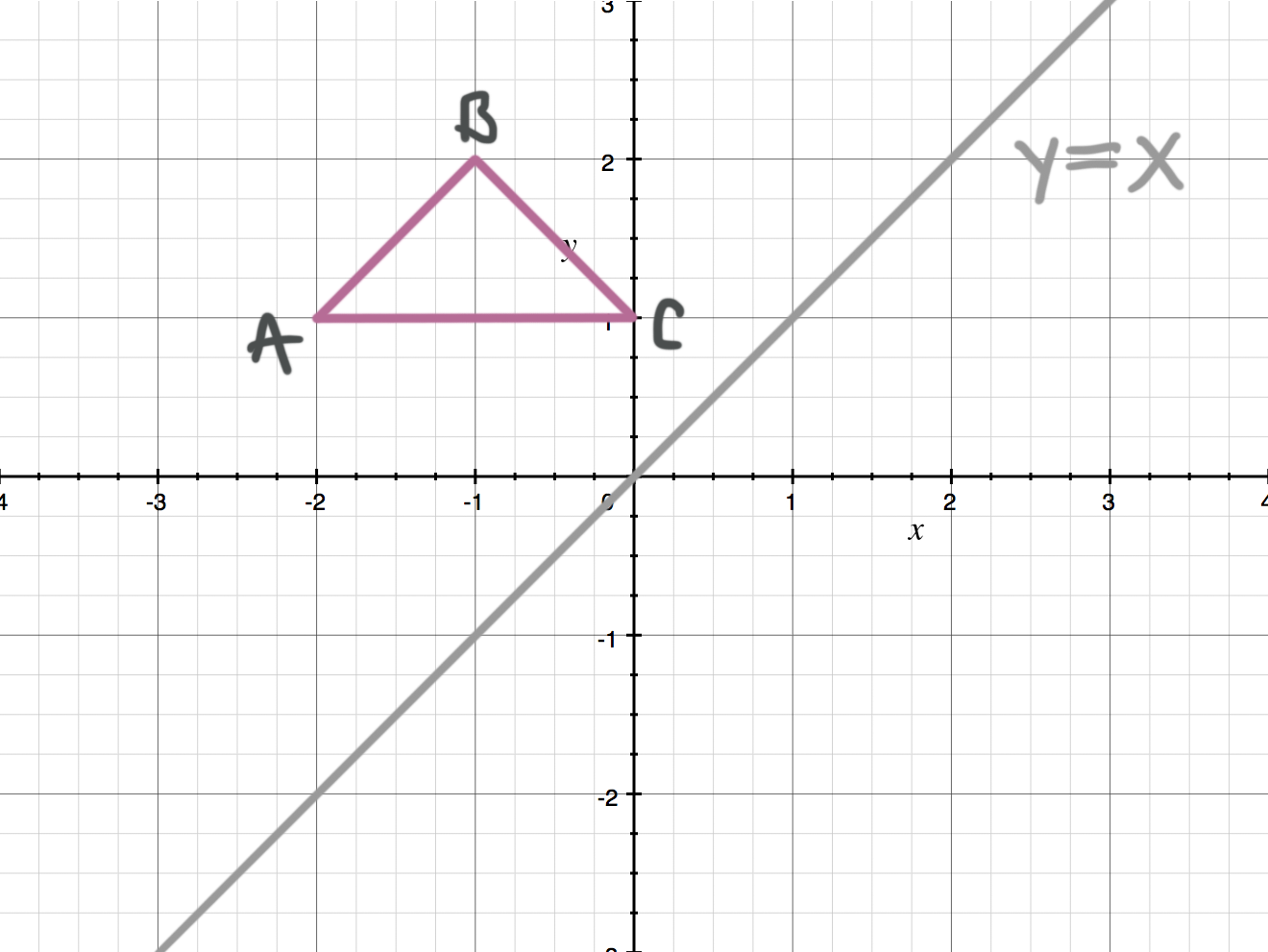



Reflecting Figures In Coordinate Space Krista King Math Online Math Tutor
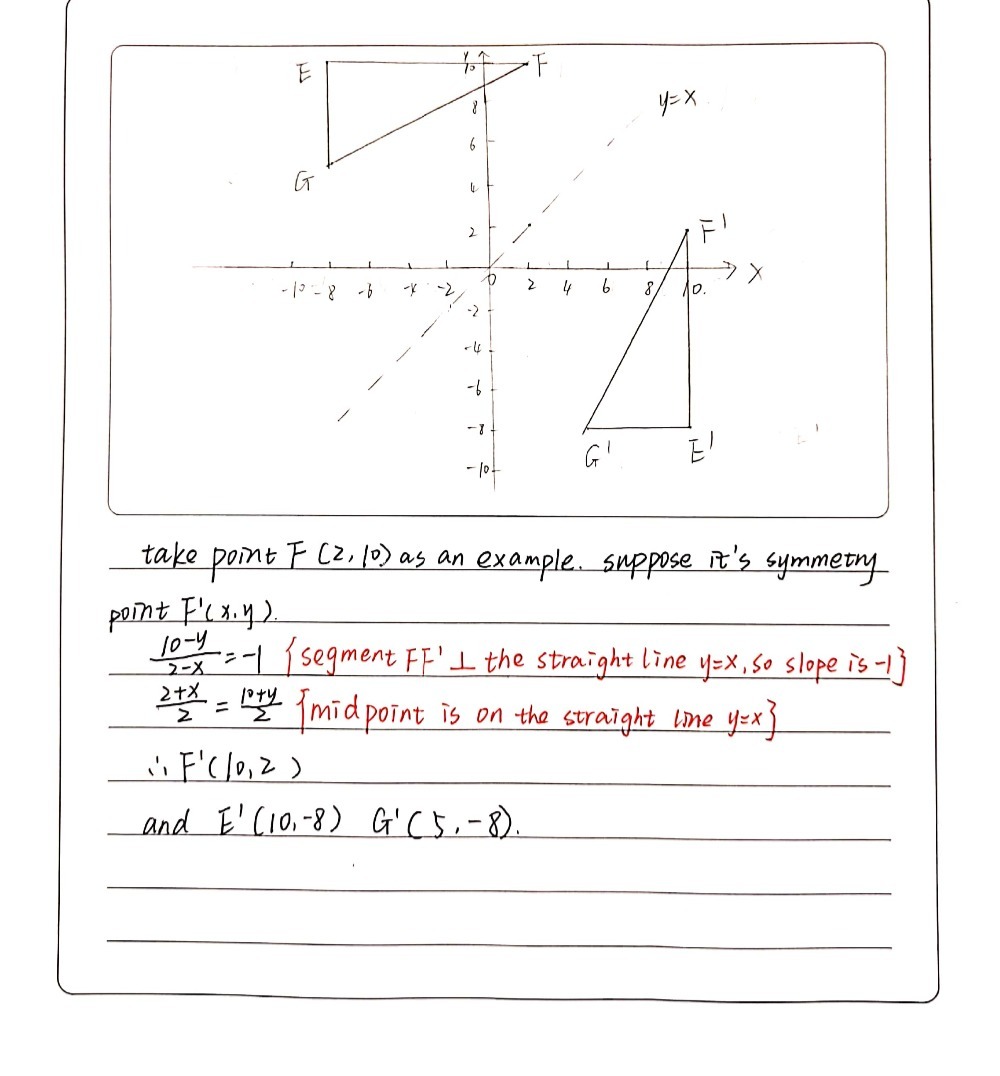



Graph The Image Of Delta Efg After A Reflection Ac Gauthmath




A Line Segment Goes From 1 2 To 4 1 The Line Segment Is Reflected Across X 1 Reflected Across Y 3 And Then Dilated About 2 2 By A Factor
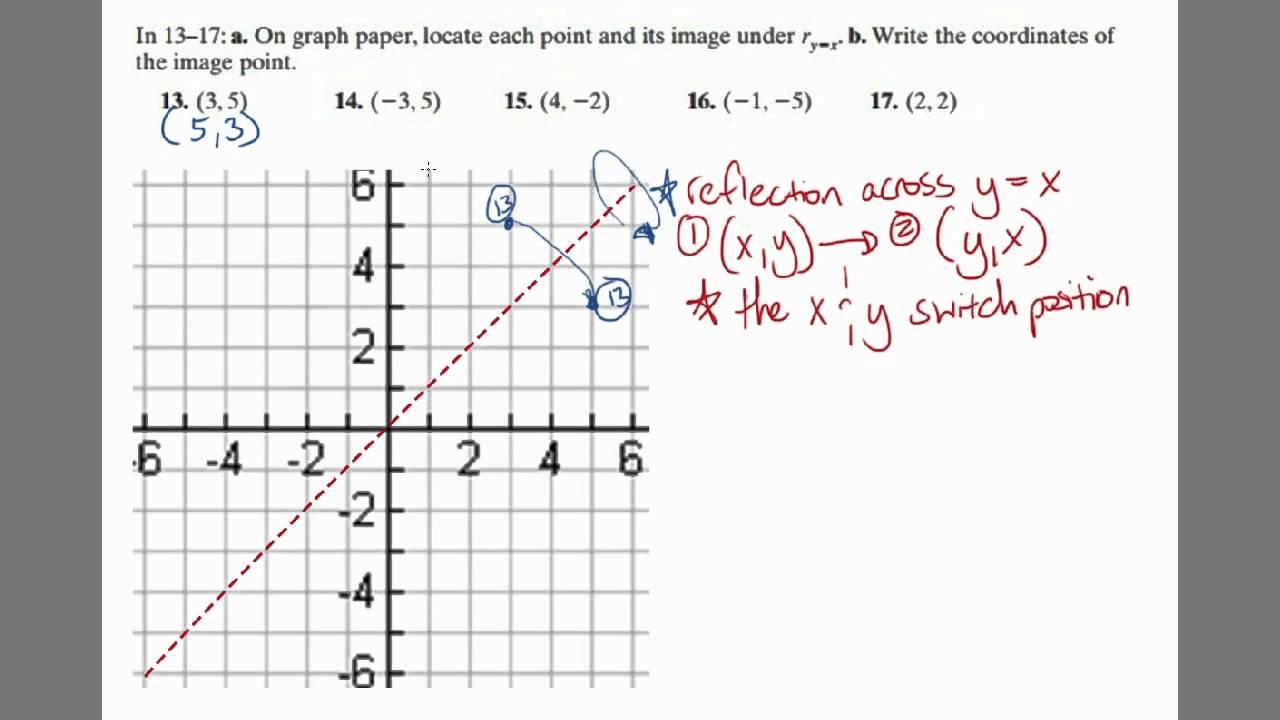



Geometry Reflection Across Y X Youtube




Which Graph Shows A Reflection Across The Line Y X Brainly Com




Introduction And Review Information Ppt Video Online Download




How To Graph Reflections Across Axes The Origin And Line Y X Video Lesson Transcript Study Com


